This "twisted tetrahedron" 
is the
simplest configuration of objects that cannot be taken apart by
translation, giving a counterexample to this conjecture. One can
prove it cannot be taken apart by checking, for each moving subset, that
every motion vector has positive projection on the normal of a moving
tetrahedron in contact with a stationary tetrahedron. This
configuration can be taken apart if we also allow rotation.
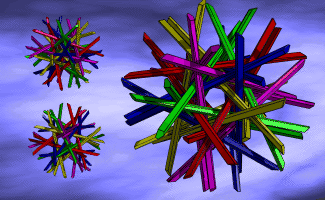However, we can nest five copies together
to form a configuration that cannot be taken apart by arbitrary rigid
motions. That is, no group of sticks can be removed without
disturbing the rest. Again, this is proved by testing, for each
possible subset (all 2 to the 30th or about 1 billion of them), that
every motion pushes a moving object into a stationary object. (An
infinitesimal motion can be represented as a vector in a six
dimensional force/torque space. Many subsets can be ruled out by
symmetries or simple geometric arguments, leaving about 120 thousand
subsets to check.)
(Here are gzipped polygon descriptions of the six-stick example (1K) and the 30-stick example (20K) in a Mathematica format (ASCII) if you'd like to experiment with them. I reserve the copyright.)